Hi David,
please help me in resolving the following confusion:
When we find correlation in CDS, I understand following :
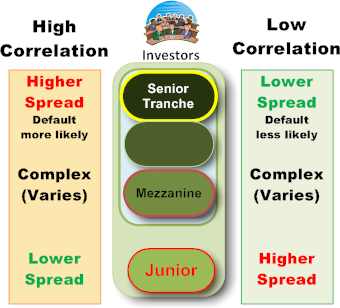
high correlation makes senior tranches (i.e., high n to default for basket CDS) more expensive (all other things equal) and junior tranches (e.g., 1st to default) less expensive;
low correlation makes senior tranches less expensive and junior tranches more expensive. You can see in my post that the binomial distribution can be used to illustrate this.
from following link
Also, In terms of payoffs of basket CDs,
More Risky will have more payoff and more price/Value
http://forum.bionicturtle.com/threads/basket-cds-value-correlation.4491/
------
So my confusion is:
in case of higher correlation, senior tranche will have more value or less value ?
A: Senior is more risk ... so more payoff/more price,
But in the following question: you said "senior tranche decreases in value due to higher correlation"
http://forum.bionicturtle.com/threads/2012-practice-exam-p2-8-cdo-tranches.5775/
I think i am missing something major here.
Should I think in terms of risk or value ?
Please help.
Thanks,
Abhi
please help me in resolving the following confusion:
When we find correlation in CDS, I understand following :
high correlation makes senior tranches (i.e., high n to default for basket CDS) more expensive (all other things equal) and junior tranches (e.g., 1st to default) less expensive;
low correlation makes senior tranches less expensive and junior tranches more expensive. You can see in my post that the binomial distribution can be used to illustrate this.
from following link

Also, In terms of payoffs of basket CDs,
More Risky will have more payoff and more price/Value
http://forum.bionicturtle.com/threads/basket-cds-value-correlation.4491/
------
So my confusion is:
in case of higher correlation, senior tranche will have more value or less value ?
A: Senior is more risk ... so more payoff/more price,
But in the following question: you said "senior tranche decreases in value due to higher correlation"
http://forum.bionicturtle.com/threads/2012-practice-exam-p2-8-cdo-tranches.5775/
I think i am missing something major here.
Should I think in terms of risk or value ?
Please help.
Thanks,
Abhi


