Learning objectives: Compute a bond’s YTM given a bond structure and price. Calculate the price of an annuity and a perpetuity. Explain the relationship between spot rates and YTM.
Questions:
906.1. Exactly one year ago, Sally purchased a $100.00 face value bond that pays a semi-annual coupon with a coupon rate of 9.0% per annum. When she purchased the bond, it had a maturity of 10.0 years and its yield to maturity (YTM; aka, yield) was 6.00%. If the bond's price today happens to be unchanged from one year ago (when she purchased the bond), which of the following is nearest to the bond's yield (yield to maturity) today?
a. 5.57%
b. 5.78%
c. 6.00%
d. 6.22%
906.2. Peter is given the opportunity to pay $100.00 in exchange for his choice of one of the following annuities:
I. The first annuity pays $2.00 per month ($24.00 per annum) over the next five years when the discount rate is 12.0% per annum with monthly compound frequency
II. The second annuity pays $18.00 per year over the next ten years when the discount rate is 12.0% annum with annual compound frequency
Both annuities pay in arrears; that is, respectively, at the end of each month and at the end of each year. Assuming Peter's discount rate of 12.0% is a flat curve (i.e., insensitive to maturity) and fully reflects his risk preferences, which of the following statements is TRUE?
a. Neither is worth the cost (the present value of both is below $100.00)
b. The monthly annuity is worth more than the annual annuity
c. The annual annuity is worth more than the monthly annuity
d. Both are worth the cost (the present value of both is above $100.00)
906.3. Assume you know that the following three spot rates, as illustrated below: 2.0% @ 0.5 years, 2.60% @ 1.0 years and 4.0% @ 1.5 years. Each of these spot rates are expressed with semi-annual compound frequency.
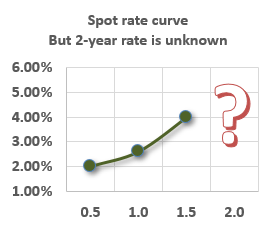
You do not know the two-year spot rate (per the red question mark above) UNTIL you informed of a bond that is trading neither cheaply nor richly but instead fairly against this spot rate term structure. This bond has a face value of $100.00 and two years to maturity; it pays a semi-annual coupon with a coupon rate of 6.0% per annum, and it offers a yield (aka, yield to maturity) of 3.770%. Given the additional information of this bond's yield, you can now infer the two-year spot rate! Which of the following is nearest to the two-year spot rate?
a. 3.80%
b. 4.10%
c. 4.49%
d. 5.00%
Answers here:
Questions:
906.1. Exactly one year ago, Sally purchased a $100.00 face value bond that pays a semi-annual coupon with a coupon rate of 9.0% per annum. When she purchased the bond, it had a maturity of 10.0 years and its yield to maturity (YTM; aka, yield) was 6.00%. If the bond's price today happens to be unchanged from one year ago (when she purchased the bond), which of the following is nearest to the bond's yield (yield to maturity) today?
a. 5.57%
b. 5.78%
c. 6.00%
d. 6.22%
906.2. Peter is given the opportunity to pay $100.00 in exchange for his choice of one of the following annuities:
I. The first annuity pays $2.00 per month ($24.00 per annum) over the next five years when the discount rate is 12.0% per annum with monthly compound frequency
II. The second annuity pays $18.00 per year over the next ten years when the discount rate is 12.0% annum with annual compound frequency
Both annuities pay in arrears; that is, respectively, at the end of each month and at the end of each year. Assuming Peter's discount rate of 12.0% is a flat curve (i.e., insensitive to maturity) and fully reflects his risk preferences, which of the following statements is TRUE?
a. Neither is worth the cost (the present value of both is below $100.00)
b. The monthly annuity is worth more than the annual annuity
c. The annual annuity is worth more than the monthly annuity
d. Both are worth the cost (the present value of both is above $100.00)
906.3. Assume you know that the following three spot rates, as illustrated below: 2.0% @ 0.5 years, 2.60% @ 1.0 years and 4.0% @ 1.5 years. Each of these spot rates are expressed with semi-annual compound frequency.

You do not know the two-year spot rate (per the red question mark above) UNTIL you informed of a bond that is trading neither cheaply nor richly but instead fairly against this spot rate term structure. This bond has a face value of $100.00 and two years to maturity; it pays a semi-annual coupon with a coupon rate of 6.0% per annum, and it offers a yield (aka, yield to maturity) of 3.770%. Given the additional information of this bond's yield, you can now infer the two-year spot rate! Which of the following is nearest to the two-year spot rate?
a. 3.80%
b. 4.10%
c. 4.49%
d. 5.00%
Answers here:
