Learning objectives: Explain the mechanics of a plain vanilla interest rate swap and compute its cash flows ... Calculate the value of a plain vanilla interest rate swap based on two simultaneous bond positions. Calculate the value of a plain vanilla interest rate swap from a sequence of forward rate agreements (FRAs).
Questions:
722.1. Consider two firms, Reliable Corp and Dubious Corp. Reliable Corp has a strong balance sheet and repayment history; it can borrow 5.0% in fixed-rate loan markets or, in floating-rate markets Reliable can pay 40 basis points above six-month LIBOR. Dubious Corp has a weaker balance sheet and must pay 7.50% in fixed-rate loan markets or, in floating-markets Dubious must pay 310 basis points above six-month LIBOR.

Which of the following statements is TRUE about this situation with respect to comparative advantage?
a. Neither has a comparative advantage in either market
b. Reliable has a comparative advantage in BOTH the fixed-rate and floating-rate markets
c. Reliable has a comparative advantage in floating-rate markets, but Dubious has a comparative advantage in fixed-rate markets
d. Reliable has a comparative advantage in fixed-rate markets, but Dubious has a comparative advantage in floating-rate markets
722.2. Suppose that some time ago a financial institution entered into a swap where it agreed to make semiannual payments at a rate of 3.0% per annum and receive LIBOR on a notional principal of $400.0 million. The swap now has a remaining life of only nine months (0.75 years). Payments will therefore be made 0.25 and 0.75 years from today. The risk-free rates with continuous compounding is assumed to be the LIBOR zero rate and currently it is 2.20% for all maturities. Because the LIBOR zero rate curve is flat at 2.20%, the six-month forward rate beginning in three months, F(0.25, 0.75), is also 2.20% with continuous compounding and therefore is equal to 2*[exp(0.0220/2)-1] = 2.2121% with semi-annual compounding. The LIBOR rate applicable to the exchange in 0.25 years was determined 0.25 years ago; suppose it was 3.0% with semiannual compounding (LIBOR has dropped in the meantime). Note: the question was inspired by Hull's Example 7.1 in his 10th edition.
Which is nearest to the present value of the swap to the financial institution?
a. -$1.550 million
b. -$287,300
c. +1.883 million
d. +2.940 million
722.3. Suppose that the 6-month, 12-month, 18-month, and 24-month overnight indexed swap (OIS) zero rates with continuous compounding are 2.00%, 2.40%, 3.00%, and 3.60%, respectively. Suppose further that the six-month LIBOR rate is 2.60% with with semiannual compounding. The forward LIBOR rate for the period between 6 and 12 months is 3.00% with semiannual compounding. The forward LIBOR rate for the period between 12 and 18 months is 3.60% with semiannual compounding. (Please Note: this question is inspired by Hull's Example 7.2 in 10th Edition).
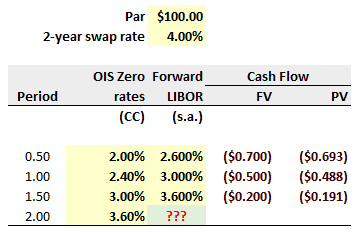
Finally and importantly, assume the two-year swap rate is 4.00%. Conditional on the realization of the LIBOR forward rates, the future cash flow in six months is therefore (2.60% - 4.00%)/2 *$100.0 = -$0.70 and its present value is about -$0.70*exp(-0.020*0.50) = -$0.693; that is, we are using the OIS zero rates as the risk-free rate for discounting purposes.
Which is nearest to an estimate for the forward LIBOR rate for the 18- to 24-month period, F(1.5, 2.0)?
a. 3.880%
b. 4.503%
c. 5.747%
d. 6.950%
Answers here:
Questions:
722.1. Consider two firms, Reliable Corp and Dubious Corp. Reliable Corp has a strong balance sheet and repayment history; it can borrow 5.0% in fixed-rate loan markets or, in floating-rate markets Reliable can pay 40 basis points above six-month LIBOR. Dubious Corp has a weaker balance sheet and must pay 7.50% in fixed-rate loan markets or, in floating-markets Dubious must pay 310 basis points above six-month LIBOR.

Which of the following statements is TRUE about this situation with respect to comparative advantage?
a. Neither has a comparative advantage in either market
b. Reliable has a comparative advantage in BOTH the fixed-rate and floating-rate markets
c. Reliable has a comparative advantage in floating-rate markets, but Dubious has a comparative advantage in fixed-rate markets
d. Reliable has a comparative advantage in fixed-rate markets, but Dubious has a comparative advantage in floating-rate markets
722.2. Suppose that some time ago a financial institution entered into a swap where it agreed to make semiannual payments at a rate of 3.0% per annum and receive LIBOR on a notional principal of $400.0 million. The swap now has a remaining life of only nine months (0.75 years). Payments will therefore be made 0.25 and 0.75 years from today. The risk-free rates with continuous compounding is assumed to be the LIBOR zero rate and currently it is 2.20% for all maturities. Because the LIBOR zero rate curve is flat at 2.20%, the six-month forward rate beginning in three months, F(0.25, 0.75), is also 2.20% with continuous compounding and therefore is equal to 2*[exp(0.0220/2)-1] = 2.2121% with semi-annual compounding. The LIBOR rate applicable to the exchange in 0.25 years was determined 0.25 years ago; suppose it was 3.0% with semiannual compounding (LIBOR has dropped in the meantime). Note: the question was inspired by Hull's Example 7.1 in his 10th edition.
Which is nearest to the present value of the swap to the financial institution?
a. -$1.550 million
b. -$287,300
c. +1.883 million
d. +2.940 million
722.3. Suppose that the 6-month, 12-month, 18-month, and 24-month overnight indexed swap (OIS) zero rates with continuous compounding are 2.00%, 2.40%, 3.00%, and 3.60%, respectively. Suppose further that the six-month LIBOR rate is 2.60% with with semiannual compounding. The forward LIBOR rate for the period between 6 and 12 months is 3.00% with semiannual compounding. The forward LIBOR rate for the period between 12 and 18 months is 3.60% with semiannual compounding. (Please Note: this question is inspired by Hull's Example 7.2 in 10th Edition).

Finally and importantly, assume the two-year swap rate is 4.00%. Conditional on the realization of the LIBOR forward rates, the future cash flow in six months is therefore (2.60% - 4.00%)/2 *$100.0 = -$0.70 and its present value is about -$0.70*exp(-0.020*0.50) = -$0.693; that is, we are using the OIS zero rates as the risk-free rate for discounting purposes.
Which is nearest to an estimate for the forward LIBOR rate for the 18- to 24-month period, F(1.5, 2.0)?
a. 3.880%
b. 4.503%
c. 5.747%
d. 6.950%
Answers here:
