Learning objectives: Define copula and describe the key properties of copulas and copula correlation. Explain tail dependence. Describe the Gaussian copula, Student’s t-copula, multivariate copula, and one-factor copula.
Questions:
707.1. Below are the joint probabilities for a cumulative bivariate normal distribution with a correlation parameter, ρ, of 0.30.
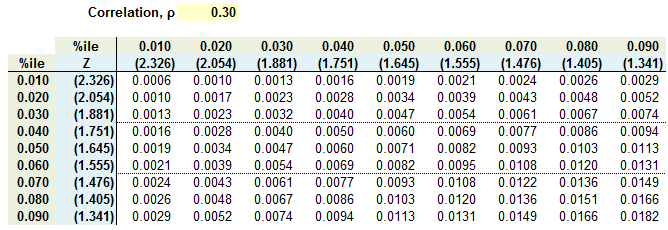
If V(1) and V(2) are each variables characterized by a uniform distribution, which is nearest to the joint probability Pr[V(1) < 0.050, V(2) < 0.050] under a Gaussian copula model?
a. 0.25%
b. 0.71%
c. 1.36%
d. 1.82%
707.2. Your colleague Roger wants to estimate the worst case default rate (WCDR) for a portfolio of retail loans. He plans to rely on Hull's expression for the worst case default rate, WCDR(T,X), which is Vasicek's application of a Gaussian copula. The audacious assumption of the model is that all loans are exposed to the same common macroeconomic factor, but this has the utter convenience of allowing Roger to estimate only the copula correlation parameter, ρ, as follows:
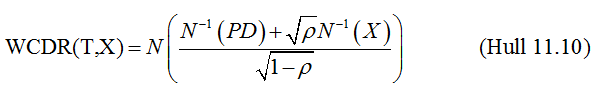
Suppose the bank has a total of $500.0 million of retail exposures of varying sizes with each exposure being small in relation to the total exposure. The one-year probability of default (PD) for each loan is assumed to be 1.250% and the loss given default (LGD) for each loan is estimated to be 64.0%. As a first draft in order to calibrate the most liberal assumption, Roger simply assumes the loans are independent such that the copula correlation parameter rho, ρ, is zero. Which is nearest to an estimate of the value at risk with a one-year time horizon and a 99.9% confidence level?
a. $4.00 million
b. $12.50 million
c. $29.23 million
d. $53.86 million
707.3. Assume two random variables, V1 and V2, are dependent and characterized by the same probability distribution but their marginal (aka, unconditional) distribution is non-normal. Let G1 and G2 represent, respectively, the cumulative unconditional probability distribution of V1 and V2; i.e., G1(v1) = Prob[V1 < v1] and G2(v2) = Prob[V2 < v2]. Each of the following statements is true EXCEPT which is false?
a. Higher tail dependence between variables V1 and V2 implies (ie, can be taken to mean) taht V2 has a higher conditional probability of an extreme upper/lower tail outcome given that V1 has an extreme upper/lower tail outcome, and vice-versa
b. A key property of a copula model is that it preserves the marginal distributions of V1 and V2, however unusual these may be, while defining a correlation structure between them, and the correlation structure can be variously defined by many different copulas
c. If we assume a Gaussian copula correlation of 0.30 in order to determine the joint probability distribution for non-normal variables V1 and V2 by mapping their marginals to bivariate normal variables U1 and U2, then this implies (by definition) that the coefficient of correlation between the non-normal V1 and V2 must be 0.30
d. If joint probability distribution F(v1, v2) is represented by a Gaussian copula then F(v1, v2)= C[G1(v1), G2(v2)] = C(u1, u2) where where C(.) is the copula function, u1 = N^(-1)[G1(v1)], u2 = N^(-1)[G2(v2)], and N^(-1) is the inverse standard normal cumulative distribution function
Answers here:
Questions:
707.1. Below are the joint probabilities for a cumulative bivariate normal distribution with a correlation parameter, ρ, of 0.30.

If V(1) and V(2) are each variables characterized by a uniform distribution, which is nearest to the joint probability Pr[V(1) < 0.050, V(2) < 0.050] under a Gaussian copula model?
a. 0.25%
b. 0.71%
c. 1.36%
d. 1.82%
707.2. Your colleague Roger wants to estimate the worst case default rate (WCDR) for a portfolio of retail loans. He plans to rely on Hull's expression for the worst case default rate, WCDR(T,X), which is Vasicek's application of a Gaussian copula. The audacious assumption of the model is that all loans are exposed to the same common macroeconomic factor, but this has the utter convenience of allowing Roger to estimate only the copula correlation parameter, ρ, as follows:
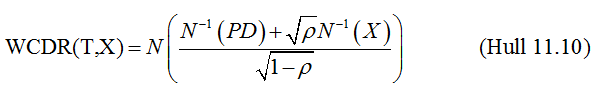
Suppose the bank has a total of $500.0 million of retail exposures of varying sizes with each exposure being small in relation to the total exposure. The one-year probability of default (PD) for each loan is assumed to be 1.250% and the loss given default (LGD) for each loan is estimated to be 64.0%. As a first draft in order to calibrate the most liberal assumption, Roger simply assumes the loans are independent such that the copula correlation parameter rho, ρ, is zero. Which is nearest to an estimate of the value at risk with a one-year time horizon and a 99.9% confidence level?
a. $4.00 million
b. $12.50 million
c. $29.23 million
d. $53.86 million
707.3. Assume two random variables, V1 and V2, are dependent and characterized by the same probability distribution but their marginal (aka, unconditional) distribution is non-normal. Let G1 and G2 represent, respectively, the cumulative unconditional probability distribution of V1 and V2; i.e., G1(v1) = Prob[V1 < v1] and G2(v2) = Prob[V2 < v2]. Each of the following statements is true EXCEPT which is false?
a. Higher tail dependence between variables V1 and V2 implies (ie, can be taken to mean) taht V2 has a higher conditional probability of an extreme upper/lower tail outcome given that V1 has an extreme upper/lower tail outcome, and vice-versa
b. A key property of a copula model is that it preserves the marginal distributions of V1 and V2, however unusual these may be, while defining a correlation structure between them, and the correlation structure can be variously defined by many different copulas
c. If we assume a Gaussian copula correlation of 0.30 in order to determine the joint probability distribution for non-normal variables V1 and V2 by mapping their marginals to bivariate normal variables U1 and U2, then this implies (by definition) that the coefficient of correlation between the non-normal V1 and V2 must be 0.30
d. If joint probability distribution F(v1, v2) is represented by a Gaussian copula then F(v1, v2)= C[G1(v1), G2(v2)] = C(u1, u2) where where C(.) is the copula function, u1 = N^(-1)[G1(v1)], u2 = N^(-1)[G2(v2)], and N^(-1) is the inverse standard normal cumulative distribution function
Answers here:
Last edited by a moderator:
