Learning objectives: Explain modern portfolio theory and interpret the Markowitz efficient frontier. Understand the derivation and components of the CAPM. Describe the assumptions underlying the CAPM. Interpret the capital market line. Apply the CAPM in calculating the expected return on an asset. Interpret beta and calculate the beta of a single asset or portfolio.
Questions:
20.8.1. The plot below illustrates an actual portfolio possibilities curve (PPC, dashed line). Two prominent portfolios (minimum variance and market portfolio) are also plotted.
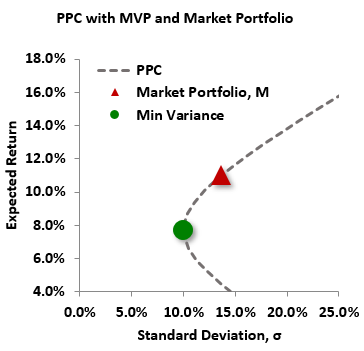
The green point is the minimum variance portfolio, MVP, that has an expected return of 7.70% and standard deviation, σ, of 10.0%. The red triangle is the Market Portfolio that has an expected return of 11.0% and standard deviation, σ, of 13.7%. If the riskfree rate is 4.0%, what is the formula for the capital market line (CML)?
a. E(R) = 0.040 + 0.511 × σ(P)
b. E(R) = 0.137 + 0.511 × σ(P)
c. E(R) = 0.040 + 0.375 × β(P,M)
d. E(R) = 0.077 + 0.511 × β(P,M)
20.8.2. Consider the market portfolio plus three other portfolios (A, B, C) with the following features while the riskfree rate is 3.0%:
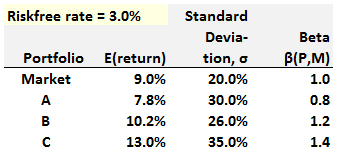
Please note the returns are expected gross returns; e.g., the market's expected return is 9.0% so that its expected excess return is 6.0%. If we assume the capital asset pricing model (CAPM) is valid then which of the following statements is TRUE?
a. Portfolio A is mispriced or it cannot exist in equilibrium due to both its beta and standard deviation
b. Portfolio B is mispriced or it cannot exist in equilibrium due to its standard deviation
c. Portfolio C is mispriced or it cannot exist in equilibrium due to its beta
d. All three portfolios can exist in equilibrium under the CAPM
20.8.3. Assume the riskfree rate is 3.0% while the market portfolio has a return of 13.0% (i.e., excess return is 10.0%) with volatility of 15.0%. Further, the following four portfolios are observed:
a. Portfolio A
b. Portfolio B
c. Portfolio C
d. Portfolio D
Answers here:
Questions:
20.8.1. The plot below illustrates an actual portfolio possibilities curve (PPC, dashed line). Two prominent portfolios (minimum variance and market portfolio) are also plotted.

The green point is the minimum variance portfolio, MVP, that has an expected return of 7.70% and standard deviation, σ, of 10.0%. The red triangle is the Market Portfolio that has an expected return of 11.0% and standard deviation, σ, of 13.7%. If the riskfree rate is 4.0%, what is the formula for the capital market line (CML)?
a. E(R) = 0.040 + 0.511 × σ(P)
b. E(R) = 0.137 + 0.511 × σ(P)
c. E(R) = 0.040 + 0.375 × β(P,M)
d. E(R) = 0.077 + 0.511 × β(P,M)
20.8.2. Consider the market portfolio plus three other portfolios (A, B, C) with the following features while the riskfree rate is 3.0%:

Please note the returns are expected gross returns; e.g., the market's expected return is 9.0% so that its expected excess return is 6.0%. If we assume the capital asset pricing model (CAPM) is valid then which of the following statements is TRUE?
a. Portfolio A is mispriced or it cannot exist in equilibrium due to both its beta and standard deviation
b. Portfolio B is mispriced or it cannot exist in equilibrium due to its standard deviation
c. Portfolio C is mispriced or it cannot exist in equilibrium due to its beta
d. All three portfolios can exist in equilibrium under the CAPM
20.8.3. Assume the riskfree rate is 3.0% while the market portfolio has a return of 13.0% (i.e., excess return is 10.0%) with volatility of 15.0%. Further, the following four portfolios are observed:
- Portfolio (A) has an expected return of 11.0% with volatility of 8.0%
- Portfolio (B) has an expected return of 7.0% with volatility of 20.0% and its correlation to the market is 0.30
- Portfolio (C) has a beta with respect to the market β(C, M) = 1.50 and its realized return of +20.0% implies an alpha of +2.0%
- Portfolio (D) has a beta with respect to the market β(C, M) = 0.40 and its realized return of +9.0% implies an alpha of +2.0%
a. Portfolio A
b. Portfolio B
c. Portfolio C
d. Portfolio D
Answers here:
Last edited by a moderator:
