Suzanne Evans
Well-Known Member
Questions:
213.1. Becky the Risk Analyst is trying to estimate the credit value at risk (CVaR) of a three-bond portfolio, where the CVaR is defined as the maximum unexpected loss at 99.0% confidence over a one-month horizon. The bonds are independent (i.e., no default correlation) and identical with a one-month forward value of $1.0 million each, a one-year cumulative default probability of 4.0%, and an assumed zero recovery rate. Which is nearest to the one-month 99.0% CVaR?
a. $989,812
b. $1.0 million
c. $1.7 million
d. $2.3 million
213.2. Assuming a credit loss distribution characterizes a portfolio of bonds or obligations, each of the following is true the credit loss distribution EXCEPT:
a. If potential losses are increasing to the right, such that x-axis is absolute|Loss| and Y-axis is f(x), the distribution will have positive skew
b. The mean of the distribution is the expected credit loss (EL) and it is generally expected that loan loss reserves should accumulate as a provision against EL and funded by (priced) additional yield as compensation for default risk
c. Increases in default correlation will not impact the portfolio's unexpected loss (UL) but will shift the portfolio expected loss (EL)
d. Portfolio UL varies with confidence, and at some (very) low confidence will correspond to a single standard deviation (one sigma) about the EL, but economic and regulatory capital will typically require multiples of (beyond) one standard deviation such that typically EC = multiplier * UL (@ 1 sigma)
213.3. A one-factor Gaussian copula model can be used to estimate the credit value at risk (CVaR) of a large portfolio of (unrealistically) similar loans. Under this model, we can be (X)% confident that the percentage of losses over a (T) year horizon will be less than V(X,T), where Q(T) is the probability of default by time (T) and rho is the copula correlation between any pair of loans:
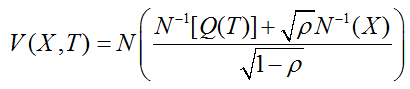
For a large retail loan portfolio, a bank wants to use this single-factor copula, which is essentially similar to the Basel II/III IRB formula for credit risk, in order to estimate a one-year 99.9% confident CVaR. The bank assumes the following:
a. $3.8 million
b. $11.0 million
c. $24.7 million
d. $30.3 million
Answers:
213.1. Becky the Risk Analyst is trying to estimate the credit value at risk (CVaR) of a three-bond portfolio, where the CVaR is defined as the maximum unexpected loss at 99.0% confidence over a one-month horizon. The bonds are independent (i.e., no default correlation) and identical with a one-month forward value of $1.0 million each, a one-year cumulative default probability of 4.0%, and an assumed zero recovery rate. Which is nearest to the one-month 99.0% CVaR?
a. $989,812
b. $1.0 million
c. $1.7 million
d. $2.3 million
213.2. Assuming a credit loss distribution characterizes a portfolio of bonds or obligations, each of the following is true the credit loss distribution EXCEPT:
a. If potential losses are increasing to the right, such that x-axis is absolute|Loss| and Y-axis is f(x), the distribution will have positive skew
b. The mean of the distribution is the expected credit loss (EL) and it is generally expected that loan loss reserves should accumulate as a provision against EL and funded by (priced) additional yield as compensation for default risk
c. Increases in default correlation will not impact the portfolio's unexpected loss (UL) but will shift the portfolio expected loss (EL)
d. Portfolio UL varies with confidence, and at some (very) low confidence will correspond to a single standard deviation (one sigma) about the EL, but economic and regulatory capital will typically require multiples of (beyond) one standard deviation such that typically EC = multiplier * UL (@ 1 sigma)
213.3. A one-factor Gaussian copula model can be used to estimate the credit value at risk (CVaR) of a large portfolio of (unrealistically) similar loans. Under this model, we can be (X)% confident that the percentage of losses over a (T) year horizon will be less than V(X,T), where Q(T) is the probability of default by time (T) and rho is the copula correlation between any pair of loans:
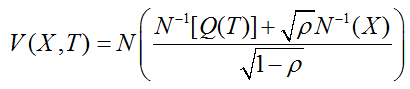
For a large retail loan portfolio, a bank wants to use this single-factor copula, which is essentially similar to the Basel II/III IRB formula for credit risk, in order to estimate a one-year 99.9% confident CVaR. The bank assumes the following:
- A total portfolio of $100.0 million consisting of many retail loan exposures,
- An average one-year default probability, Q(T), of 1.0%
- A copula correlation parameter, rho, of 0.25
- A recovery rate of 40.0%
a. $3.8 million
b. $11.0 million
c. $24.7 million
d. $30.3 million
Answers:
