P1.T3.510. Dollar roll (Tuckman)

[Nicole Seaman]

Learning outcome: Describe a dollar roll transaction and how to value a dollar roll.

Questions:

510.1. Consider an investor who wants to finance the purchase of a mortgage pool over a one month period. One alternative is to sell an MBS repo, in which case the investor could sell the pool today while simultaneously agreeing to repurchase it after a month. This trade has the same economics as a secured loan: the investor effectively borrows cash today by posting the pool as collateral, and, upon paying back the loan with interest after a month, retrieves the collateral. An alternative is the "dollar roll." In the dollar roll, the buyer of the roll sells a TBA for one settlement month (the "earlier month") and buys the same TBA for the following settlement month (the "later month").

For example, the investor who just purchased a 30-year 4% FNMA pool might sell the FNMA 30-year 4% January TBA and buy the FNMA 30-year 4% February TBA. Delivering the pool just purchased through the sale of the January TBA, which raises cash, and purchasing a pool through the February TBA, which returns cash, is very close to the economics of a secured loan.

But there are two important differences between dollar roll and repo financing:

I. The buyer of the roll may not get back in the later month the same pool delivered in the earlier month. The buyer of the roll delivers a particular pool, for example, in January but will have to accept whatever eligible pool is delivered in the next February. By contrast, an MBS repo seller is always returned the same pool that was originally posted as collateral.

II. The buyer of the roll does not receive any interest or principal payments from the pool over the roll. For example, the buyer of the Jan/Feb roll, who delivers the pool in January, does not receive the January payments of interest and principal. By contrast, a repo seller receives any payments of interest and principal over the life of the repo. While the prices of TBA contracts reflect the timing of payments, so that the buyer of a roll does not, in any sense, lose a month of payments relative to a repo seller, the risks of the two transactions are different. The buyer of a roll does not have any exposure to prepayments over the month being higher or lower than what had been implied by TBA prices while the repo seller does.

Which of these two differences is (are) correct?

a. Neither are correct
b. I. is true but II. is incorrect
c. I. is incorrect but II. is true
d. Both are correct


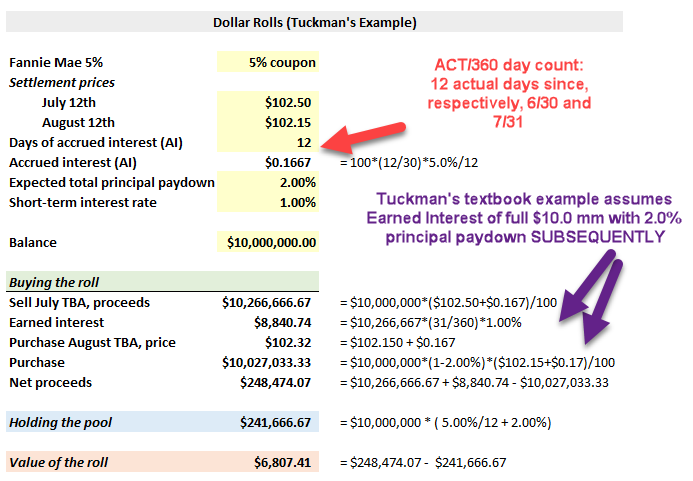
510.2. Consider the scenario (a variation on Tuckman's example) illustrated below. Suppose that the TBA prices of the Fannie Mae 6% for July 9 and August 9 settlements are $103.00 and $102.60, respectively. The accrued interest to be added to each of these prices is 9 actual/360 days of a month's worth of a 6.0% coupon, i.e., 100 × (9/30) × 6.0%/12 or $0.150. Let the expected total principal paydown, that is, scheduled principal plus prepayments, be 2.0% of outstanding balance and let the appropriate short-term rate be 1.0%.

If an investor rolls a balance of $10.0 million, proceeds from selling the July TBA are $10.0 million × (103.00 + 0.150)/100 or $10,315,000.00. Investing these proceeds to August 9 at 1.0% earns interest of $10,315,000.00 × (31/360) × 1.0% or $8,882.36. Then, purchasing the August TBA, which has experienced a 2.0% principal paydown, costs $10.0 million × (1 - 2.0%) × (102.60 + 0.150)/100 or $10,069,500.00. The net proceeds from the roll, therefore, are $10,315,000.00 + $8,882.36 - $10,069,500.00 or $254.382.36. If the investor does not roll, the net proceeds are the coupon plus principal paydown: $10,000,000.00 × (6.0%/12 + 2.0%) or $250,000.00.
(Tuckman's example: Bruce Tuckman, Angel Serrat, Fixed Income Securities: Tools for Today’s Markets, 3rd Edition (New York: Wiley, 2011))

Given this scenario, which of the following is true?

a. The roll trades above carry (i.e., the value of the roll is positive) and this might be rationally explained by delivery options of TBAs
b. The roll trades above carry (i.e., the value of the roll is positive) and this might be rationally explained by the relatively low short-term interest rate
c. The roll trades below carry (i.e., the value of the roll is negative) and this might be rationally explained by delivery options of TBAs
d. The roll trades at breakeven (i.e., the value of the roll is zero) and this is expected in efficient markets


510.3. Which of the following is most likely to exhibit a dollar value of an 01 (DV01) that is negative?

a. Principal-only (PO) at high yields
b. Interest-only (IO) strip at low yields
c. Interest only (IO) strip at high yields
d. Planned amortization class (PAC) bond at low yields

Answers here:

• In forum

 

[Taunk]

Hi,
In 502.2 , the last line of the question (which is also in the study notes) states "If the investor does not roll, the net proceeds are the coupon plus principal paydown: $10,000,000.00 × (6.0%/12 + 2.0%) or $250,000.00".

I have a very rudimentary doubt that if the investor does not roll, then why are we adding the additional 2% as he should only be entitled to receive the stated coupon.

Thanks,

 

[Deepak Chitnis]

Hi @taunk, as per question, scheduled principal plus prepayments, be 2.0% of outstanding balance additional 2% is just outstanding balance. Hope that helps. Thank you!

 

[Taunk]

Thanks Deepak. But i am afraid that i am not able to grasp the point .

 

[David Harper CFA FRM]

Hi @taunk

Both scenarios (ie, buying the roll and holding the pool) contain $200,000 which is the 2.0% (of $10.0 million) expected total principal paydown, as @Deepak Chitnis explains. It's a cash flow perspective, not a fully-loaded yield or something like that. The pool will experience principal paydown, which is actual cash. In the case of holding the pool, the cash flow over the month includes the interest and the principal paydown. In the case of buying the roll, please note the purchase price ($10,069,500) is reduced by the same $200,000, so it's consistently in both scenarios. Thanks,

To a related question about the 2.0%, here is my answer here @ https://forum.bionicturtle.com/threads/p1-t3-502-dollar-roll-tuckman.8494/#post-35212

Hi Melody (@superpocoyo ) Yes, my questions uses 2.0% just like Tuckman's example (emphasis mine): "To make the roll more concrete, consider the following example. Suppose that the TBA prices of the Fannie Mae 5% for July 12 and August 12 settlements are $102.50 and $102.15, respectively. The accrued interest to be added to each of these prices is 12 actual/360 days of a month's worth of a 5% coupon, i.e., 100 × (12/30) × 5%/12 or .167. Let the expected total principal paydown, that is, scheduled principal plus prepayments, be 2% of outstanding balance and let the appropriate short-term rate be 1%." -- Tuckman, Bruce; Serrat, Angel (2011-10-11). Fixed Income Securities: Tools for Today's Markets (Wiley Finance) (Kindle Locations 13917-13921). Wiley. Kindle Edition.

In my example, the 2.0% is (also) expected total principal paydown which therefore figures into both scenarios:

• If the investor "rolls the balance," the August Purchase = $10,069,500.00 = $10,000,000 (original) balance * (1 - 2% principal paydown) * ($102.60 + $0.150 accrued interest)/100; i.e., the pool has experienced a 2.0% principal paydown.
• Compared to holding the pool = $250,000 = $10,000,000 * (6.0% coupon/12 + 2.0% principal paydown). I hope that explains!

 

[Taunk]

Thanks David & Deepak. It is clear now.

 

[Angelinelyt]

Hi! Please can i ask how is this equation derived from question 2

100 × (9/30) × 6.0%/12 or $0.150.

 

[David Harper CFA FRM]

Hi @Angelinelyt My questions models Tuckman's own example, so I copied below his example (emphasis mine to highlight the parallel assumption). Please note that the coupon is 6.0% per annum such that the monthly coupon is 6.0%/12 per month. But we don't accrue a full month, we are only accruing 9 days out of a 30-day month (assumed), so we want (9/30) of the monthly coupon, which is (9/30)*(6.0%/12), which is a percentage (%), so we multiply that by the face value to get the dollar amount: $100* (9/30)*(6.0%/12) = $100 face * (9 days /30 day month )*(6.0% per annum /12 months ) = $0.150. I hope that explains! Tuckman's example is below:

"In market jargon, the value of the roll is the difference in proceeds between 1) starting with a given pool and buying the roll and 2) holding that pool over the month. If the value of the roll is zero, as it would be if the forward pricing methodology of Chapter 13 applied, the roll is said to trade at breakeven. If the forward drop is larger so that the value of the roll is positive, the roll is said to trade above carry. Given the delivery option of TBAs, the roll would be expected to trade somewhat above carry without necessarily implying a value opportunity.

To make the roll more concrete, consider the following example. Suppose that the TBA prices of the Fannie Mae 5% for July 12 and August 12 settlements are $102.50 and $102.15, respectively. The accrued interest to be added to each of these prices is 12 actual/360 days of a month's worth of a 5% coupon, i.e., 100 × (12/30) × 5%/12 or .167. Let the expected total principal paydown, that is, scheduled principal plus prepayments, be 2% of outstanding balance and let the appropriate short-term rate be 1%.

If an investor rolls a balance of $10 million, proceeds from selling the July TBA are $10mm × (102.50 + .167)/100 or $10,266,700. Investing these proceeds to August 12 at 1% earns interest of $10,266,700 × (31/360) × 1% or $8,841. Then, purchasing the August TBA, which has experienced a 2% principal paydown, costs $10mm × (1–2%) × (102.15 + .167)/100 or $10,027,066. The net proceeds from the role, therefore, are $10,266,700 + $8,841 − $10,027,066 or $248,475.

If the investor does not roll, the net proceeds are the coupon plus principal paydown, i.e., $10mm × (5%/12 + 2%) or $241,667. In conclusion, then, the roll is trading above carry in this example, with the value of the roll at $248,475 − $241,667 or $6,808." -- Tuckman, Bruce; Serrat, Angel. Fixed Income Securities: Tools for Today's Markets (Wiley Finance) (p. 574). Wiley. Kindle Edition.

 

[Bucephalus]

Hi @David Harper CFA FRM I have a couple of questions

1) On the accrued interest calculation during the "re-purchase" in August. Will the principal pay down of 2% happen on August 9? What I want to understand is that if the principal paydown happens earlier, the accrued interest in August will not amount to 100 × (9/30) × 6.0%/12 or $0.150, but rather (1-0.02)*100 × (9/30) × 6.0%/12 (changes depending on when the pay down happens)

2) The earned interest is calculated for 31 days (which is fine as jul has 31 days). But, when the accrued interest is calculated, we have 9/30. Is it because of the 30/360 convention (I was under the impression that 30/360 convention is applicable only to bonds).

 

[David Harper CFA FRM]

Hi @Bucephalus Those are good points!

1. I do appreciate that the timing of principal re- and pre-payment matters in these models, but to be honest, I do not know the dollar roll rules. Instead, I can only infer from Tuckman's own modelling assumptions (which I believe I followed). Here is the XLS (and corresponding snapshot below) in which I replicated Tuckman's own dollar roll example: https://www.dropbox.com/s/r0e08084nkwfpag/0821-tuckman-dollar-roll.xlsx?dl=0 ... To your point, the Earned Interest that accrues to the Net Proceeds is taken on the full balance; and subsequently the 2.0% Expected Principal Paydown assumption "leaks" the August purchase. I infer the 2.0% paydown occurs--in the model--between July 12th and August 12th. Although it seems to me that the key feature is that the 2.0% paydown is treated consistently between the two scenarios ("buying the roll" versus "holding the roll"), which it is. Put another way, if we decrease (increase) the Expected Total Principal Assumption, then both (Buying scenario's) Net proceeds and Holding the pool decrease (increase). And I notice varying this has quite an impact on the Value of the Roll. (Of course, Tuckman could be modeling this assumption incorrectly, we've identified other errors: I am only using his example to infer the answer to your question! But in this case, I don't have experience/resource to contradict the assumption)
2. No, I don't think that 30/360 applies here. Tuckman does state, at least in the example, that the day count convention is ACTUAL/360 ("To make the roll more concrete, consider the following example. Suppose that the TBA prices of the Fannie Mae 5% for July 12 and August 12 settlements are $102.50 and $102.15, respectively. The accrued interest to be added to each of these prices is 12 actual/360 days of a month's worth of a 5% coupon ..."). However, we might expect the ACT/360 money market convention to apply here, right? But notice that in terms of sell July, it really is actually 12 days from 6/30 (i.e., end of month) to 7/12 and, with respect to the August purchase, it is 12 actual days from 7/31 (i.e., end of month) to 8/12. Similarly, in my 510.2, I used July 9th (ie, 9 actual days since the end/beginning of the month) and August 9th (9 actual days since the end/beginning of month).